1. If \( f(x)=-x^2+4, -1\leq x \leq 3 \), evaluate the Riemann sum with \( n=4\), taking the sample points to be right endpoints.

Solution

2. The graph of \(g\) is shown. Estimate \( \displaystyle \int_{-1}^{4}g(x)\, dx \) with five intervals using left endpoints.




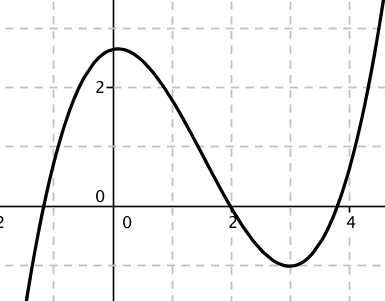
Solution

3. The graph of \( f\) consists of straight lines and a semicircle. Use the given graph to evaluate the following.


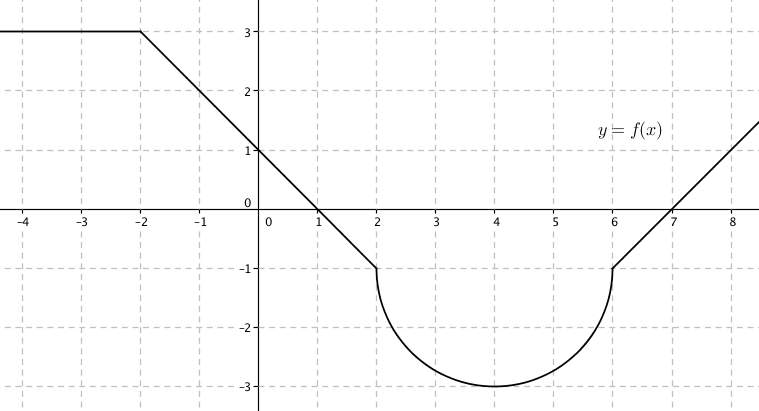
(a) \( \displaystyle \int_{-4}^{-1} f(x) dx \)

Solution

(b) \( \displaystyle \int_{-4}^{1} f(x) dx \)

Solution

(c) \( \displaystyle \int_{2}^{6} f(x) dx \)

Solution

(d) \( \displaystyle \int_{6}^{6} f(x) dx \)

Solution

(e) \( \displaystyle \int_{6}^{2} f(x) dx \)

Solution

(f) \( \displaystyle \int_{-4}^{6} f(x) dx \)

Solution

(g) If \( \displaystyle g(x) =\int_{0}^{x} f(t) dt \), evaluate \( g(8) \).

Solution

4. If \( \displaystyle \int_{-3}^{10} f(x)\;\; dx = 20 \) and ,\( \displaystyle \int_5^{-3} f(x) \;\; dx = 7\) , find \( \displaystyle \int_{10}^{5} f(x) dx \). Solution